Adaptive polynomial chaos expansions for uncertainty quantification and sensitivity analysis in structural dynamics

Uncertainty is ubiquitous in the design and analysis of engineering structures and systems. Advanced methods for representing the sources of uncertainty and propagating them through computational models are necessary for designing safe and robust structures under cost constraints. In this context, polynomial chaos (PC) expansions have emerged as a powerful tool for propagating the parameter uncertainties through the computational models and help solve typical questions of safety (“what is the probability of failure of the system?”), sensitivity analysis (“what are the important parameters that drive the system behaviour?”) and decision-making (“what is the best scenario / compromise between cost and safety?”).

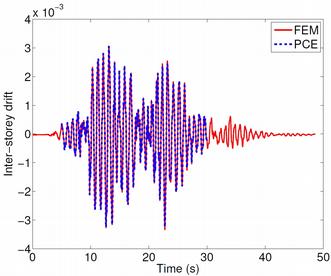
In continuity with the work by Blatman & Sudret [1,2], this research project investigates new fields of application of polynomial chaos expansions, namely dynamics problems in which the model response is a time history. The envisaged application is the robust computation of fragility curves of buildings in earthquake engineering.

In order to be applicable to the assessment of realistic structures, a PC expansion procedure has to be parsimonious in terms of the number of runs of the computer model. For this purpose, new local error estimates are developed in order to devise a fully adaptive procedure. Starting from a small set of model runs (so-called initial experimental design), the next model runs to be carried out are defined so as to maximize the information gained. Applications in structural dynamics as well as geotechnics are envisaged.

References

[1] Blatman, G. and Sudret, B., Adaptive sparse polynomial chaos expansion based on Least Angle Regression, J. Comput. Phys 230 (2011) 2345-2367.

[2] Blatman, G. and Sudret, B., Efficient computation of global sensitivity indices using sparse polynomial chaos expansions, Reliab. Eng. Sys. Safety 95 (2010) 1216-1229